Intersection of two Circles

The intersection points of two circles and their connecting line are calculated.

Example 1:

Given are the circles :         
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯ 
k1 : M1(5|5) r1 = 5
k2 : M2(0|0) r2 = 5

Intersection points :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯ 
S1(5|0)   S2(0|5)

Connecting line :
¯¯¯¯¯¯¯¯¯¯¯¯¯
 x + y = 5

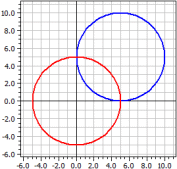
Example 2:

Given are the circles :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  k1 : M1(5|5)   r1 = 5/sqrt(2)
  k2 : M2(0|0)   r2 = 5/sqrt(2)

Point of tangency:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  B(2,5|2,5)

Tangent line:
¯¯¯¯¯¯¯¯¯¯
 x + y = 5

See also:

Setting the graphics